Margaret Gaida on Genres of Astrological Texts for Teaching and Learning

Written on September 14th, 2021 by Margaret Gaida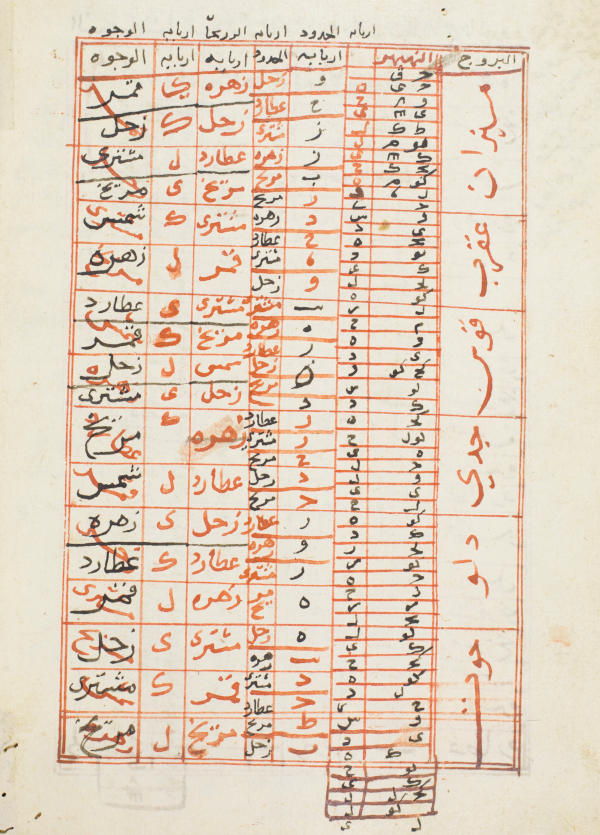

In considering the history of the occult sciences in Islamic contexts, there is still much work to be done in (re)examining materials associated with the “hard” sciences, especially astronomy, including astrolabes and planetary tables. As much occult content was overlooked or dismissed by previous historians, there is much to be gained from this reexamination and engagement. In this case, several introductory astrological texts point to the importance of planetary tables, or zijes, for pedagogical purposes.

Between the ninth and eleventh centuries, several introductory texts on astrology appeared across the Islamic world. These included several with substantial historical footprints, including al-Qabīṣī’s Kitāb al‐mudkhal ilā ṣināʿat aḥkām al‐nujūm, originally composed in 960 CE and extant in over 25 Arabic manuscripts, as well as over 230 Latin manuscripts and other European vernacular translations, and printed up until the year 1560. The Introduction provides an explanation of the basic principles of astrology, that is, qualitative descriptions of the influences of the planets given their position in the sky with respect to other celestial bodies and divisions and to an observer’s location on earth. It includes details about the properties of the zodiac, descriptions of the astrological houses, and the properties of the planets, both in themselves and in relation to other planets and parts of the sky. Planets and their positions both hold astrological significance. One might also expect to find instructions for astrological techniques, such as producing a horoscope.

However, in reading through al-Qabīṣī’s descriptions of basic astrological principles, technical explanations are notably absent, including things like the calculations of the house cusps, which is necessary for most astrological inquiries. Instead, the technical content usually involves very basic arithmetical calculations related to establishing qualitative parameters. The text is by no means what we would consider a handbook or technical manual. But this is not due to al-Qabīṣī’s technical incompetence. He also composed treatises on the sizes and distances of the planets and a commentary on al-Farghānī’s Elements of Astronomy. So, how did students of astrology actually learn the techniques behind making astrological prognostications?

Let’s consider more carefully the genre of the mudkhal, or Introduction, within the astrological tradition. As mentioned, several introductory texts of this genre appeared in the ninth, tenth, and eleventh centuries. Here I will be primarily focusing on al-Qabīṣī and Kūshyār ibn Labbān’s introductory texts. These texts assimilated and synthesized astrological knowledge stemming from Greek, Syriac, and Persian sources, relying especially on the major Greek astrological treatises, Ptolemy’s Tetrabiblos and Dorotheos of Sidon’s Book on Nativities. The introductions also provided basic preparation for understanding the specialized treatises on astrological subjects such as nativities, historical astrology, elections, and interrogations by the late eighth/early ninth-century authors Māshā’allāh, Sahl ibn Bishr, and Umar Ibn Farrukhān al-Tabarī. As with al-Qabīṣī, the early introductions are comprised of an explanation of the interpretive framework of astrology, with few technical instructions for making calculations.

In terms of the importance of these texts for astrological pedagogy, several authors justify the need for introductory texts in their prefaces. The Mudkhal of al-Qabīṣī, for example, explicitly addresses the pedagogical necessity of a concise synthesis of astrological tenets: “When I looked at all the astrologers of the past who composed books which they called ‘introductions’ (mudākhil) to this craft, and some did not examine in the (books) everything that should have been in an introduction, and some were too prolix, bringing in things that were not necessary for (an introduction so that) the necessary things disappeared, and some did not follow the right order of instruction in their arrangement of the material, I composed this book and put it together as an introduction, collecting in it, from the sayings of (my) predecessors, all that is needed for the craft, following the method of an introduction.”¹ He is no doubt addressing his immediate predecessor Abū Maʿshar al-Balkhī, whose expansive Kitāb al-Mudkhal al-Kabīr gives a justification of astrology within the context of Aristotelian natural philosophy. The Abbreviation (al-Mukhtasar) of the Great Introduction is much more similar to al-Qabīṣī’s Mudkhal but is arranged somewhat differently. Al-Qabīṣī is thus attentive to a pedagogical methodology within the genre of a mudkhal, and we may indeed consider his text as pedagogically innovative.

One example of an innovation that al-Qabīṣī introduced in his Mudkhal is an entire chapter on “the explanation of the (technical) terms of the astrologers” (tafsīr samāt al-munajimīn). This chapter provides explanations of several important calculations and determinations, including the haylāj, intiha (terminal point), and tasyīr. Some of these points are qualitatively determined based solely on their position in the sky, rather than by performing a calculation. The haylāj, often translated as the governor or indicator, for nativities, for example, involves a process of elimination based on the time of birth. Al-Qabīṣī explains the elaborate process for determining the haylāj, beginning first with the location of the Sun in the sky and its astrological house, and whether it falls under a masculine or feminine sign. Continuing through a series of conditions, the astrologer then considers whether the Moon, degree of conjunction or opposition prior to the birth, Lot of Fortune, or degree of the ascendant could be suitable for the haylāj. The determination is straightforward if the celestial conditions at birth are well-established, and does not involve any quantitative calculation.

However, in the discussion of the haylāj there is a reference to the determination of the house cusps, since determining the haylāj involves a consideration of the astrological houses: “Concerning the haylāj you look in the cardines and the succedents according to (the way that) the 12 places are equalized by the time degrees of ascension, (namely,) according to what has been explained in the Zij.”² The determination of the haylāj depends on precisely where the haylāj falls within the houses, which depends upon how the houses are divided. Without a zij at hand and a consistent method for dividing the houses, this calculation would be impossible.

The haylāj also plays a key role in the determination of the tasyīr, or prorogator, which is used for calculating the length of life. The difference in degrees between the haylāj and another point, once converted into solar years, provides the length of life and is known as the tasyīr. Al-Qabīṣī’s descriptions of the calculation of the tasyīr do not mention the zij explicitly, but his language definitely implies that students would be making use of a zij. The calculation of the tasyīr involves several preliminary calculations which demand values of right and oblique ascension which one would find in a zij. Rather than giving a descriptive astronomical method for this calculation, al-Qabīṣī provides arithmetical instructions based on parameters found in the zij. For example, he writes: “Pertaining to this is the tasyīr (prorogation). This is that you move an indicator to a position on the ecliptic, and you (want to) know what is (the distance) between the two in equatorial degrees—that is, what is rotated of the time-degrees of the equator with regard to the position from which it is moved until it is in the position to which it is moved. One takes a year for each degree. If you want this and the indicator which you want to move to a degree of the ecliptic is in the ascendant, you subtract the rising-times of the degree of that which you want to move from the rising-times of the degree to which you want to move it. What remains are the degrees of the motion (tasyīr).”³ There is no real explanation for a method of tasyīr computation beyond the consultation of tables. Also of note here is his use of the imperative to instruct his readers, which raises the question of how much the Mudkhal was intended for use as an auto-didactical text and underscores the pedagogical approach of al-Qabīṣī.

Written at the end of the tenth century, Kūshyār ibn Labbān’s introduction expands on the descriptions of al-Qabīṣī. Kūshyār provides different circumstances of tasyīr calculations and gives several examples. However, he also includes two tables of tasyīr calculations, one based on a specific example and the other on the calculation of a monthly tasyīr. In addition to the tables, he makes several references to the use of zijes, especially his own. In his discussion of the monthly tasyīr he writes: “the operation in this becomes easy because of the tables of the mean (motion) of the Sun in the Zij.”⁴ He subsequently references another explanation for tasyīr computation in his zij: “If we understand the revolution we make its computation. Its operations are based on what we have explained in the Zij.”⁵

Another chapter, “On the Calculation of Prorogators (tasyīrāt)” repeats many of the same arithmetical calculations based on conditions of the indicator that we found in al-Qabīṣī. This section is analagous to al-Qabīṣī’s descriptions of the arithmetical calculations once the tasyīr parameters are known. Kūshyār begins the section by proclaiming: “This chapter belongs better to a Zij, and we have mentioned it there. We intend to mention it in this book so that it might be comprehensive when it is needed.”⁶

In considering the introductions themselves, we may conclude that the lack of technical content and the references to zijes in the introductions of al-Qabīṣī and Kūshyār ibn Labbān reveal that students of astrology from the ninth to eleventh centuries would have made frequent use of zijes for the parameters related to astrological technical calculations. However, the introductions also reference explanations for particular calculations, such as the determination of the house cusps.

In E.S. Kennedy’s survey of Islamic astronomical tables, he documents “astrological tables,” and gives several examples of these: the determination of the house cusps, the projection of the rays, tables for year-transfers (when the sun crosses the vernal equinoctial point) or nativity-transfers (determined for each year when the sun crosses the same zodiacal point that it occupied at the moment of birth), and tables of progressions, which we know as the tasyīr. The angular relationships between planets—or planetary aspects—was also an important factor in prognostication. Some zījes describe various methods of planetary influence, or rays emanating from and impacting other planets, known as the projection of the rays. In al-Maj̲rīṭī’s recension (c. 1000 CE) of the famous zīj composed by al-Khwārizmī in 825 CE, there is a lengthy table devoted to the projection of the rays, which takes up more space than any of the other tables in the zīj.

A later section in several zijes takes up more specific topics of mathematical astrology, such as those mentioned above (year transfers, nativity transfers, progressions, projection of the rays) as well as Saturn-Jupiter conjunctions, and lunar mansions. Several zījes, for example the zīj of Ḥabash al-Ḥāsib (c. 850) give tables for year-transfers and nativity-transfers. The former is the moment when the sun traverses the vernal equinox; the latter refers to the instant every year when the sun enters the same zodiacal point as the moment of birth. Zījes also contain tables for the excess of revolution, or the excess of the true solar year over 365 days, expressed in equatorial degrees. The Mumtaḥan zīj of Yāḥya ibn Abī Manṣūr (c. 810 CE), contains a table with a set of values for the excess of revolution found in other zījes. Other topics one may find in zījes are Saturn-Jupiter conjunctions and lunar mansions. It is also important to note that tables may have an astrological function that is not immediately obvious to someone unfamiliar with astrological calculations. We wouldn’t find a reference to the tasyīr, for example, next to the tables of right and oblique ascension.

In terms of how students learned astrology, and particularly its technical content, it is difficult to generalize from the textual evidence alone, particularly across the expansive Islamic empire and the waxing and waning in the popularity of astrology across centuries. In the early period, especially the ninth to eleventh centuries, it appears that students of astrology would have used the zīj to learn how to make calculations, accompanied by a mudkhal to understand the significance of these calculations and how to go about interpreting them. It is likely that learning astrology with the use of these texts would have been facilitated by a practicing astrologer, who would no doubt have supplemented the textual material with oral instruction. More work needs to be done in documenting and assessing the astrological content of zijes. We may conclude, at least, that introductory astrological texts and planetary tables formed a pedagogical couplet that served as the basis for astrological learning.

Author bio

Margaret Gaida is currently a Postdoctoral Researcher at Caltech. Her research focuses on the transmission and exchange of astronomical and astrological knowledge between Europe and the Islamic world during the period between 900 and 1600 CE. Dr. Gaida is interested in the materiality of texts, textual genres, and the intersection of texts, images and instruments. Her work has been funded by the American Academy in Rome, the Council for American Overseas Research Centers, and the American Council of Learned Societies. Dr. Gaida is currently working on her first book, The Lost Art of Arabic Astrology.

IOSOTR

Margaret Gaida on Genres of Astrological Texts for Teaching and Learning

Author bio

Further Reading

Margaret Gaida on Genres of Astrological Texts for Teaching and Learning

Author bio

Further Reading

See also...

Łukasz Piątak on Astral Worship in al-Suhrawardī’s al-Wāridāt wa'l-taqdīsāt

Elizabeth Sartell on Ways of Knowing in Ibn al-ʿArabī's Story of Creation